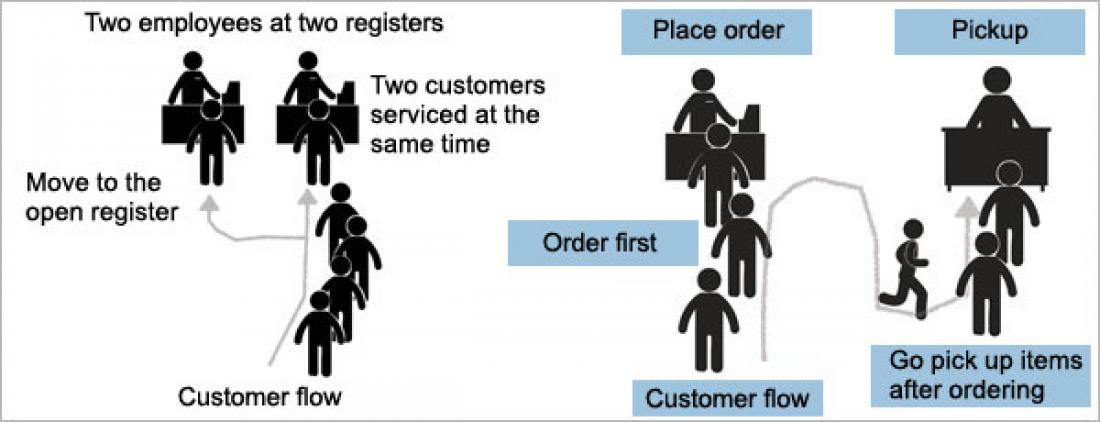
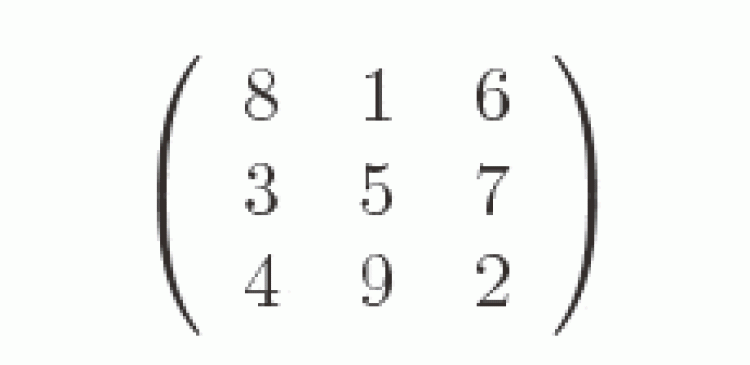Figure 1. Two-employee queueing style at McDonald’s (left) vs. queueing style at Starbucks (right) (Reprinted from [1].)
You’ve probably already noticed the difference between the way people line up at McDonald’s and the way they line up at Starbucks. At McDonald’s, a single employee handles your entire order. Even if we’re left wondering whether the person is new or wishing they would move a little faster, we are able to relax knowing that our Big Mac is on its way. At Starbucks, once we order and pay, we’re told that our items will be ready at the far counter. Though we’re all pretty much used to it by now, we wait anxiously, wondering whether someone else is going to pick up our caramel latte. Psychological factors aside, let’s take a look at whether one queueing style is more efficient than the other (figure 1), or whether McDonald’s and Starbucks are the same in that regard.
In Japanese, we use the same two Chinese characters to refer to a waiting line or queue and to a mathematical matrix. In mathematics, a matrix is typically a set of numbers arranged in rows and columns, like the one you see below. (This matrix happens to be a special one. Can you guess why?)
Numbers that are “lined up” are called a matrix, but I’d like to talk about a different kind of lineup—where people arrange themselves in a waiting line, or queue. We learn in economics that prices change when there is an imbalance between supply and demand. Creating a queue is an easy method of handling a situation where demand temporarily exceeds supply. When there are more customers in the store than there are hamburgers being made and sold, McDonald’s doesn’t raise the price of its burgers, it instead has the customers form a line at the counter. When increases in demand are temporary, lines will soon disappear. Researchers have been fascinated by this phenomenon for a very long time, causing them to enthusiastically study its features and characteristics.
We encounter queues in all kinds of everyday situations—sitting in traffic, at a popular noodle shop, or when the latest smartphone is launched. Personally, I happen to be a huge tennis fan, and travel to England to watch the Wimbledon Championships. Tennis enthusiasts from across the globe seeking same-day tickets come to stand in the most famous waiting line in the world, known simply as The Queue (figure 2). Though The Queue is an exception in that it is actually dear to the hearts of tennis lovers, most people have an aversion to queues because they are forced to wait to receive a product or service. Even I despise lines, and I study them!
Let’s take a closer look at the lines we dislike so much. At McDonald’s, a single employee takes the orders and delivers the items at each register. At Starbucks, the ordering and pickup counters are separate, which results in two separate queues. Let’s say we have a situation like figure 1, where the two employees have exactly the same skill level. Since the McDonald’s employees handle the work from beginning to end, it seems like we are waiting a long time to get our order. However, if dividing up the tasks does not make the work more efficient, the McDonald’s approach is better. Why? Starbucks divides its customers into two lines—so when one of the lines disappears, the employee in charge of that line is left with nothing to do. This doesn’t happen at McDonald’s, since there is a single queue in front of the two employees. We can therefore mathematically prove that on average, the waiting times are longer in a Starbucks queue. There is a detailed discussion of this in my work cited below [1]. Naturally, there are times when dividing up the workload is more efficient, and it is possible that this approach offers the benefit of allowing one employee to focus on making coffee well, for example, so we can’t categorically say that Starbucks’ way of doing things is wrong. (My apologies to Starbucks—I added some supplementary comments.)
We learn all kinds of interesting things when we look at commonplace occurrences through the lens of mathematics and science.
[Works Cited]
[1] Shigeo Shioda, Kenichi Kawanishi, Hiroshi Toyoizumi, and Masaki Aida, Into the Future with Digital Series 29 Queueing Theory: Fundamentals and Applications [Mirai he Tsunagu Dejitaru Shirizu 29 Machi Gyoretsu Riron no Kiso to Oyo], Kyoritsu Shuppan, 2014.
ABOUT THE AUTHOR
Hiroshi Toyoizumi
Professor, Graduate School of Accountancy, Waseda University
[Profile]
March 1989: Completed Master’s Program in Pure and Applied Physics at the Faculty of Science and Engineering, Waseda University
March 1998: Received Doctorate in Engineering from the Faculty of Science and Engineering, Waseda University
April 1989: Began working at Nippon Telegraph and Telephone Corporation
June 1999: Appointed Lecturer at the Performance Evaluation Lab, the University of Aizu
April 2005: Appointed assistant professor at the Graduate School of Accountancy, Waseda University
April 2007 to present: Joint appointment to the Department of Applied Mathematics, Waseda University
April 2009 to present: Appointed professor at the Graduate School of Accountancy, Waseda University
July 2013 to present: Appointed expert advisor for the National Public Service Examination
Awards
March 1997: 1996 Young Researcher Award, Institute of Electronics, Information and Communication Engineers
March 1999: 1998 SSE Research Award from the Exchange Research Committee, Institute of Electronics, Information and Communication Engineers
March 2013: BIOTECHNO 2013 Best Paper Award
Major Publications
Queueing Theory: Fundamentals and Applications [Machi Gyoretsu Riron no Kiso to Oyo] (co-author, Kyoritsu Shuppan, 2014).
The Monte Carlo Method Handbook [Montekaruro-ho Handobukku] (co-author, Asakura Publishing, 2014).
Society Memberships
The Operations Research Society of Japan, The Institute of Electronics, Information and Communication Engineers, The Japan Society for Industrial and Applied Mathematics, IEEE, INFORMS, SIAM, Japanese Society for Mathematical Biology